Trusses are composed of thin structural members that are in compression or tension. Since there are only two joints in a given member, only two forces can act on the member. This means all members are two-force members and thus the member load acts in the direction of the two pins. If the member is straight (no curvature) then there is no bending moment in the member.

When modeling trusses, it is assumed that

1. all loads are applied at joints
2. all joints are pins, and support no moment

Load in Two-Force Member Acts
in the Direction of the Joints

Method of Joints

Each Joint Must be in Equilibrium

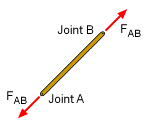
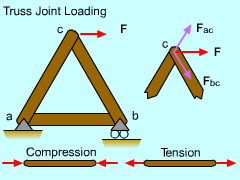
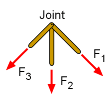
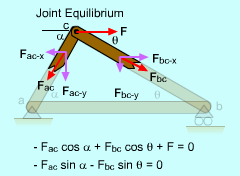
One of the basic methods to determine loads in individual truss members is called the Method of Joints. Like the name states, the analysis is based on joints. Each joint is treated as a separate object and a free-body diagram is constructed for the joint.

Because each and every joint must be in equilibrium, the basic force equations can be applied to each joint,

     ΣF_x = 0
     ΣF_y = 0

Each joint will only have two equations to solve for member forces since there is no moment at the joint. The means only two unknown member forces can be solved at a single joint and the order in which the joints are solved is important.

Care must be taken in drawing force vectors. A compression member will 'push' the joint, but a tension member will 'pull' the joint.