| Ch 6. Structures | Multimedia Engineering Statics | ||||||
| 2-D Trusses: Joints | 2-D Trusses: Sections | 3-D Trusses | Frames and Machines | ||||
| 2-D Trusses: Method of Joints | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Example |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||
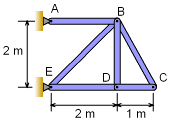 Truss Supporting its own Weight |
Determine the force in each member due to the weight of the truss. Assume the weight of the members are uniform along their length and have a mass density ρ of 9 kg/m. (g = 9.81 m/s2) |
||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Solution |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||
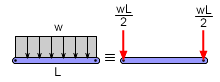 Equivalent Loading for Truss Member |
The only loading on the truss is the weight of each member. Since all loads on a truss must act at the joints, the distributed weight of each member must be split between the two joints. Assume the weight of each member is a vertical force, half of which is placed at each end of the member as shown in the diagram on the left. The weight of each member is |
||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
WAB = ρgLAB = 9 (9.81) 2 = 176.6 N WBD = WED = WAB = 176.6 N WEB = ρgLEB WBC = ρgLBC WDC = ρgLDC |
||||||||||||||||||||||||||||||||||||||||||||||||||||||
The weight of each member is considered as an external force on the end points. The external force at each joint is half of the weight of the bar. The table below summarizes how the weight of each member is distributed to its own joints. The table also gives the total force at each joint.
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
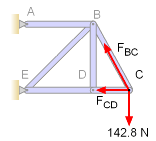
Now that the total load for all the joints are know, each joint can be analyzed. Joint C is a good point to start with, since there are only two unknown member forces, FBC and FCD. The summation of forces in y direction for the joint C gives, ΣFy = 0 The summation of the forces in x direction gives, ΣFx = 0 |
||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
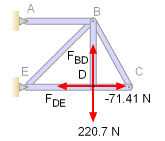
Joint D ΣFx = 0 ΣFy=0 |
||||||||||||||||||||||||||||||||||||||||||||||||||||||
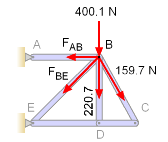 Joint D |
Joint B, ΣFy= 0 ΣFx=0 Notice, the boundary reactions at A and E were not needed. This is unusual and is due to the structure being cantilevered. Generally, reactions need to be determined first, then member forces. |
||||||||||||||||||||||||||||||||||||||||||||||||||||||