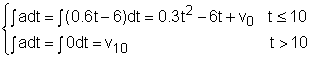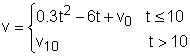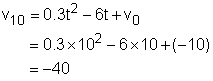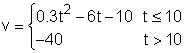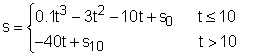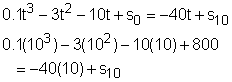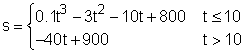| Ch 6. Integrals | Multimedia Engineering Math | ||||||
|
Indefinite Integral |
Area |
Definite Integral |
Fundamental Theorem |
Substitution Rule |
|||
| Indefinite Integral | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Limits |
| 2. Derivatives I |
| 3. Derivatives II |
| 4. Mean Value |
| 5. Curve Sketching |
| 6. Integrals |
| 7. Inverse Functions |
| 8. Integration Tech. |
| 9. Integrate App. |
| 10. Parametric Eqs. |
| 11. Polar Coord. |
| 12. Series |
| Appendix |
| Basic Math |
| Units |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
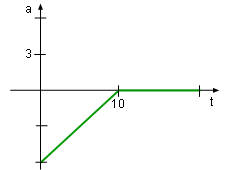 Acceleration Over Time |
A water droplet drops from 800 m above the ground with an initial speed 10 m/s. Its acceleration is expressed as How long does it take for the droplet to hit the ground? Assume the upward direction is positive and let the time, velocity and distance be t, v and s respectively. The velocity at 0 amd 10 s is represented as v0 and v10 respectively. Likewise, s0 and s10 are the upward distance at 0 and 10 s. |
|
 Driblet |
In order to calculate the time for the droplet to reach the ground, it is necessary to find its distance function. The velocity is the derivative of the distance and the acceleration is the derivative of the velocity. In other words, the velocity is the integral of the acceleration and the distance is the integral of the velocity. One way to solve this problem is to integral the acceleration two times to find its distance function. Integrating the acceleration with respect to time t gives the velocity function. |
|
Simplifing the velocity is The initial velocity of the droplet is given, v0 equals -10 m/s. Next, substituting t = 10 into the above equations gives v10. The final velocity is |
||
Integrating the velocity with respect to time t gives the distance function. Therefore, the function of the distance is The droplet is dropped from 800 m, so s0 is 800. Substituting t = 10 and s0 = 800 into the distance function gives the value of s10. Rearranging this equation gives s10 = 900 Substituting s0 = 800 and s10 = 900 into the distance function gives |
||
When the droplet reaches the ground, the distance is 0 and thus -40t + 900 = 0 or t = 22.5 s |
||