| Ch 6. Rigid Body Force and Acceleration | Multimedia Engineering Dynamics | ||||||
|
Moment of Inertia |
Translational Motion | Fixed Point Rotation |
General Plane Motion |
||||
| Moment of Inertia | Case Intro | Theory | Case Solution | Example |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
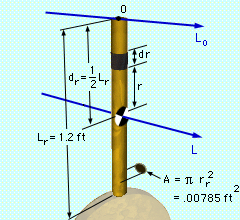 Dimensions |
First find the moment of inertia of the slender rod about a line L passing through its center of mass. "Slender" means the length is much greater than the width or depth. Consider a differential element of length dr at a distance r from the center of mass. The mass of the element is equal to the product of its density and volume: dm = ρ dv = ρ A dr Substitute this into equation for the moment of inertia of the rod about L, Note that the mass of the rod is given by mr = ρ Vrod = ρ A Lr thus Irod = 1/12 mr Lr2 This is the same result listed in the Sections Appendix. for a slender rod. |
|
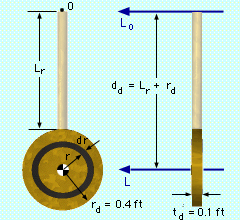 Dimensions |
Now the parallel axis theorem can be used to calculate the moment of inertia of the rod about the line Lo passing through the point 0, Io-rod = Irod + dr2 mr = 1/12 mr Lr2 + (1/2 Lr)2 mr = 1/3 mr Lr2 = 0.3333 (0.5 slugs) (1.2 ft)2 = 0.2400 slugs-ft2 Next, determine the moment of inertia of the disk about a line L passing through its center of mass, perpendicular to the flat face of the disk. Consider a differential element of width dr at a radius r from the center of mass. The mass of the element is equal to the product of its density and volume: dm = ρ dv = ρ td 2 π r dr Substitute this into the moment of inertia equation to get I of the disk about L, = 0.5 ρ td π rd4 The mass of the element is equal to the product of its density and volume, md = ρ Vd = ρ π rd2 td Idisk = 1/2 md rd2 Again, parallel axis theorem can be used to find the moment of inertia of the disk about the line Lo passing through the point 0, Io-disk = Idisk + dd2 md = 1/2 md rd2 + (Lr + rd)2 md = md [1/2 rd2 + (Lr + rd)2] = 2.67 [1/2 (0.4)2 + (1.2 + 0.4)2] = 7.049 slugs-ft2 |
|
The sum of the moment of inertia of the disk and rod to will give the total moment of inertia of the pendulum about Lo as Io-pendulum = Io-rod + Io-disk = 7.289 slugs-ft2 |
||
Practice Homework and Test problems now available in the 'Eng Dynamics' mobile app
Includes over 400 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.
