| Ch 5. Rigid Body Equilibrium | Multimedia Engineering Statics | ||||||
|
2-D and 3-D Supports |
Equilibrium in 2-D |
Equilibrium in 3-D |
Indeterminate Objects | 2 and 3 Force Members | |||
| Equilibrium in 3-D | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Introduction |
||
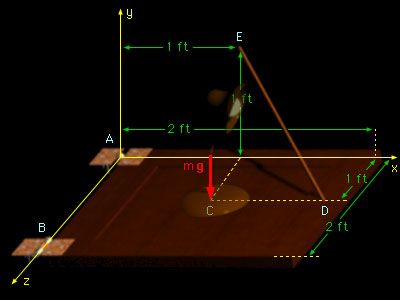
 Problem Description Graphic |
As Louis the Lamp is making his great escape from the lonely attic, he must jump onto the trap door that will set him free. Before he leaps, however, he must know the tension he will place on the rope that supports the door. What is known:
|
|
|
|
||
| Questions |
||
|
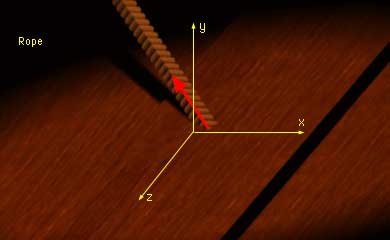
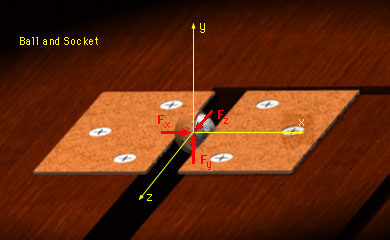
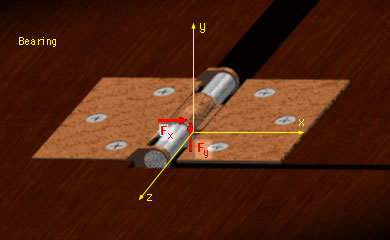
What are the reaction components at each of the supports? What is the tension in the rope? |
||
| Approach |
||
|
||
|
|
||
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.