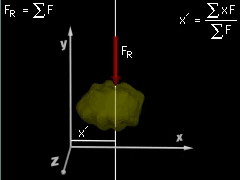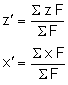| Ch 4. Moments/Equivalent Systems | Multimedia Engineering Statics | ||||||
|
Moment 2-D Scalar |
Moment 3-D Scalar |
Moment 3-D Vector |
Couples and Equiv. System | Distributed Loads, Intro | |||
| Introduction to Distributed Loads | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
|
|
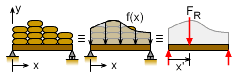
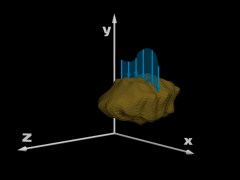
In many static problems, applied loads are given as distributed force loads. This is similar to stacking sand bags on a beam so that the load is distributed across the beam instead of at one location (point load). To help make the problem easier to solve, it is convenient to convert the distributed load into equivalent point loads. |
|
| Discrete Distributed Force |
||
|
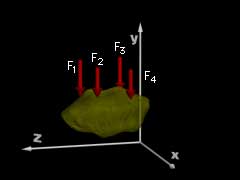
If the loading on the object is a set of parallel discrete forces, the resultant force is simply the sum of all the forces, or FR = ΣFi In the previous section, three scalar equations were derived that determine the position r of a force FR that represents the force resultant to a system of discrete forces. If all forces are parallel to the y direction then the three scalar equations simplify to Σ(zF) = z' ΣF Σ(xF) = x' ΣF Here x and z are the Cartesian coordinates of the individual forces, and x' and z' are the coordinates of the force resultant FR. Rearranging these two equations gives If the forces are further restricted so that they all lie in the x-y plane, then z' = 0 and only the second equation applies. |
|
 Distributed Forces
|
||
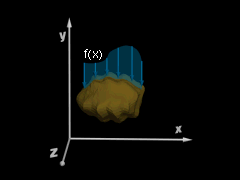
| Continuous Distributed Force |
||
|
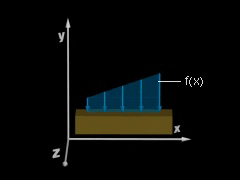
If instead of a system of point loads, consider a continuous distributed force f(x) that acts in the x-y plane and is parallel to the y axis, then through calculus the second equation (x') above becomes The force resultant is simply the force magnitude FR given by The force magnitude FR is located a distance x' from the origin.
|
||
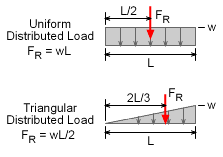
| Uniform and Triangular Line Loads |
||
|
|
In the case of a uniform line load as shown, it is unnecessary to perform the integrations because the force resultant is always the value of the distributed load multiplied by the distance over which it acts. The location of the force resultant is always the center point (centroid) of the distributed load. For a triangular line load, it can be shown that the force resultant is one half of the peak value of the distributed load multiplied by the distance over which it acts. The location of the force resultant is two-thirds of the distance from the vertex to the peak value of the load. |
|
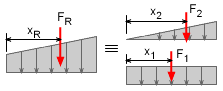
 Composite Line Load
|
Composite Loading |
|
|
A load of the type shown is said to be a composite load since it can be generated with a combination of simpler loads such as a uniform and a triangular line load. Loads of this nature can be converted to force resultants by splitting the load into its composite parts, solving for the force resultant of each part, and then combining the forces into a force resultant for the entire load. As an example, the diagram at the left can be splitting to a triangular and rectangular distributed load. The two separate loadings can be combined as, FR = F1 + F2 xR FR = x1 F1 + x2 F2 |
||
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.