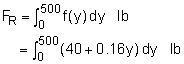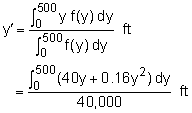| Ch 4. Moments/Equivalent Systems | Multimedia Engineering Statics | ||||||
|
Moment 2-D Scalar |
Moment 3-D Scalar |
Moment 3-D Vector |
Couples and Equiv. System | Distributed Loads, Intro | |||
| Introduction to Distributed Loads | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Integration Method |
||
 Integration Method
|
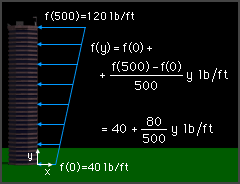
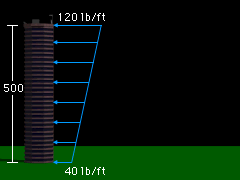
In order to integrate the distributed wind load, determine a function f(y) that describes the wind load. f(y) = 40 + 80/500 y lb/ft The force resultant is given by Its location is given by |
|
| Composite Parts Method |
||
|
|
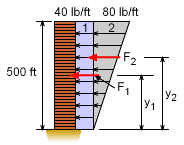
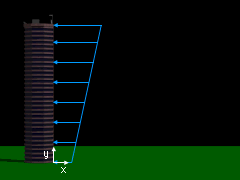
For the composite parts method, first break the wind load
into two parts: a uniform load of 40 lb/ft and a triangular load
of 0 lb/ft at the base and 80 lb/ft at the top of the building.
The force resultant of the uniform load and its location is F1 = 40 (500) = 20,000 lb y1 = 250 ft The force resultant of the triangular load is F2 = 0.5 (80) (500) = 20,000 lb Its location is two-thirds of the distance from the vertex to the peak value y2 = 2/3 (500) = 333.3 ft Nest, combine these two forces into a single force FR located at the position y'. FR = ΣF = F1 + F2 = 40,000 lb Its location is given by Substituting F1, y1, F2, and y2 and simplifying the equation gives y' = 1.166 x 107 / 40,000 |
|
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.