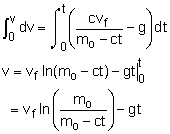| Ch 4. Particle Momentum and Impulse | Multimedia Engineering Dynamics | ||||||
| Impulse & Momentum | Consv. Linear Momentum | Impact |
Angular Momentum |
Mass Flow |
|||
| Mass Flow | Case Intro | Theory | Case Solution | Example |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
|
|
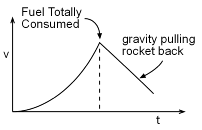
Use Newton's Second Law to sum all the forces acting on the rocket and equate it to the acceleration times mass, ΣF = ma Ff - mg = m (dv/dt) The thrust, Ff, is due to the mass flow of the burning propellant. Using the mass flow equation gives, Ff = -(dmf /dt) vf = (dmf /dt) vfj = cvfj Notice, all motion and forces are only in the y direction. Combining equations for the y-direction, cvf - mg = m (dv/dt) Rearrange to get dv = (cvf /m - g)dt At any time t, the total mass of the rocket and the remaining fuel can be expressed as m = mo - (dmf /dt) t = mo - ct By substituting, the change in velocity, dv, is |
|
|
|
Integration will give an expression for the velocity as The total time required to burn all the fuel is mf = (dmf /dt)ttot = c ttot ttot = mf /c Now that the time needed to burn all the fuel is known, it can be substituted back into the velocity equation to give, where vf = fuel velocity (constant) |
|
Practice Homework and Test problems now available in the 'Eng Dynamics' mobile app
Includes over 400 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.