Dynamics eBook: Impact

Ch 4. Particle Momentum and Impulse					Multimedia Engineering Dynamics
Impulse & Momentum	Consv. Linear Momentum	Impact	Angular Momentum	Mass Flow

DYNAMICS - EXAMPLE
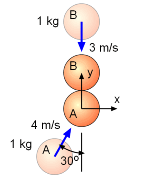Collision of Two Balls		Example
		Two balls, A and B, impact each other as shown. If the coefficient of restitution, e, is 1.0 (perfectly elastic), what is the velocity magnitude of ball B after impact?

		Solution
Velocity of Balls Before and After Collision		Ball B only has a velocity in the y-direction, so first consider y-direction momentum. Conservation of momentum before and after impact gives m_Av_A1y + m_Bv_B1y = m_Av_A2y + m_Bv_B2y 4 cos30 - 3 = -v_A2y + v_B2y 0.4641 = -v_A2y + v_B2y There are still unknowns, so the impact equation needs to be used, e = (v_B2y - v_A2y)/(v_A1y - v_B1y) 1 = [v_B2y - (-v_A2y)] / [4 cos30 - (-3)] 3.464 + 3 = v_B2y + v_A2y 6.464 = v_B2y + v_A2y Combining both equations 0.4641 = -(6.464 - v_B2y) + v_B2y 0.4641 + 6.464 = 2v_B2y v_B2y = 3.464 m/s v_B2y = v_B2 = 3.464 m/s

Example Problem 1


Example Problem 2


Example Problem 3


Example Problem 4


Example Problem 5


Example Problem 6


Example Problem 7


Example Problem 8


Example Problem 9


Example Problem 10


Example Problem 11


Example Problem 12


Example Problem 13


Example Problem 14


Example Problem 15


Example Problem 16


Example Problem 17


Example Problem 18


Example Problem 19


Example Problem 20


Example Problem 21