| Ch 4. Particle Momentum and Impulse | Multimedia Engineering Dynamics | ||||||
| Impulse & Momentum | Consv. Linear Momentum | Impact |
Angular Momentum |
Mass Flow |
|||
| Conservation of Linear Momentum | Case Intro | Theory | Case Solution | Example |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
|
|
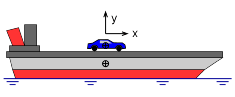
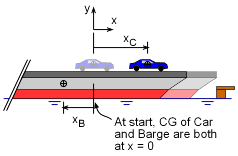
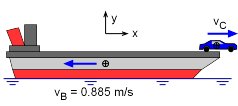
Begin with a diagram of the car and barge in their initial state. Place the origin of the rectangular coordinate system at the initial position of the car. The velocity of the barge when the car reaches the end of the barge is vB = -0.885i m/s Because the horizontal force of the water on the barge is neglected, the total linear momentum in the horizontal direction is conserved. |
|
| Velocity |
||
 Velocity of Barge and Car as Car Leaves Barge |
Since the car and barge are initially stationary, the total linear momentum is initially zero. mCvC + mBvB = constant = 0 The velocity of the car at the end of the barge can now be solved as vC = -mB/mC vB = -(2,500/500)(-0.885)i m/s = 4.425i m/s |
|
| Position |
||
|
|
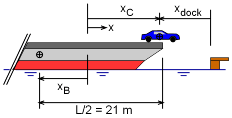
The combined CG of the car and the barge is initially stationary, so it must remain stationary. Knowing the position of the combined CG, the position of the car and the barge relative to their original positions can be determined. The diagram at the left shows the position after motion starts. The initial combined rCG is equal to zero since that is the location of the coordinate system. 0 = mC xC - mB xB There are still two unknowns, xC and XB. However, when the car leaves the barge, the combined distance xB and xC must be half the barge distance, xC + xB = 0.5 L Combining with the previous equation gives, 0 = mC xC - mB (0.5L - xC) 0 = (500 + 2,500)xC - 2,500 [0.5 (42)] xc = 17.5 m The distance from the dock is xdock = 21 - 17.5 xdock = 3.5 m |
|
Practice Homework and Test problems now available in the 'Eng Dynamics' mobile app
Includes over 400 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.