| Ch 4. Beam Stresses | Multimedia Engineering Mechanics | ||||||
|
Bending Strain and Stress |
Beam Design |
Shear Stress |
Built-up Beams |
||||
| Bending Strain and Stress | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Stress/Strain |
| 2. Torsion |
| 3. Beam Shr/Moment |
| 4. Beam Stresses |
| 5. Beam Deflections |
| 6. Beam-Advanced |
| 7. Stress Analysis |
| 8. Strain Analysis |
| 9. Columns |
| Appendix |
| Basic Math |
| Units |
| Basic Mechanics Eqs |
| Sections |
| Material Properties |
| Structural Shapes |
| Beam Equations |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Example |
||
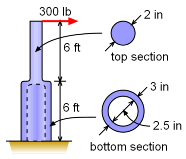 Fixed Pole with Load |
A 12 foot pole rigidly fixed to the ground is loaded with a 300 lb horizontal force at its top. The bottom half of the pole is hollow and the half is solid, as shown in the diagram on the left. What is the maximum bending stress at any location in the pole? |
|
| Solution |
||
|
|
The maximum bending stress is a direct function of the maximum bending moment and the member cross section, as given by, σ = My / I Since there are two different cross sections, both will need to be checked. |
|
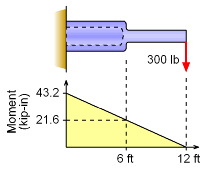 Free-Body Diagram |
First, the moment distribution from the bottom to the top of the pole needs to be determined so that the maximum can be identified. The pole can be considered as a simple cantilevered beam which makes finding the moment diagram simple. If a cut is made at an arbitrary location, x, then the moment at that location is Maa =
(300 lb) (12 - x ft)(12 in/ft) The maximum in the bottom section is 43.2 kip-in at the ground. The maximum for the top section is 21.6 kip-in at the mid-point joint. The moment of inertia for both sections are, Itop = πd4/64 = π24/32 = 0.7854 in4 Ibottom = π/64 (34 - 2.54) = 2.059 in4 |
|
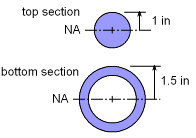 Pipe Cross Sections |
The stress in both sections are σtop-max = My / I
= 21.6(1)/0.7854 The maximum stress occurs in the bottom section. |
|
Practice Homework and Test problems now available in the 'Eng Mechanics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.