| Ch 3. Particle Energy Methods | Multimedia Engineering Dynamics | ||||||
|
Work & Energy |
Conservative Forces | Power & Efficiency | |||||
| Conserv. Forces/ Potential Energy | Case Intro | Theory | Case Solution | Example |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| |
|||||
| Conservation of Energy |
|||||
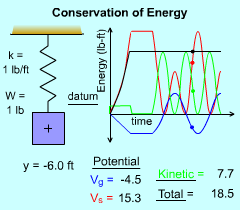 Conservation of Energy for a Spring-Mass System |
In the previous section, Work and Energy was developed for a non-conservative system. However, in many problems there are only conservative forces which permits an alternative way to develop the energy balance equation. A conservative force means that the work done by the force is independent of the path. Gravity and springs are conservative forces, but friction is not. If there are only conservative forces, then the total energy at any point in time (or position) must be constant. Generally, conservative forces are grouped as potential (V) or kinetic (T) energy. V1 + T1 = constant If the total energy is constant, then the total energy at one point must equal the total energy at another point in the motion.
At any given time, all energy in a conservative system is defined as kinetic or potential energy. |
||||
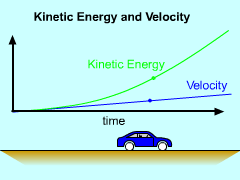 Kinetic Energy and Velocity |
|||||
| Kinetic (motion) Energy |
|||||
| Kinetic energy is based on the velocity of the object. Recall from the previous section, T = ½mv2
|
|||||
| Potential (stored) Energy |
|||||
 Potential Energy |
Energy that can be stored and converted to kinetic energy is referred to as potential energy, Gravity Potential Vg = mgy Spring Potential Vs = ½ks2
|
||||
Practice Homework and Test problems now available in the 'Eng Dynamics' mobile app
Includes over 400 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.