| Ch 3. Derivatives II | Multimedia Engineering Math | ||||||
|
Higher Order Derivatives |
Related Rates |
Differential |
Newton's Method |
||||
| Higher Order Derivatives | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Limits |
| 2. Derivatives I |
| 3. Derivatives II |
| 4. Mean Value |
| 5. Curve Sketching |
| 6. Integrals |
| 7. Inverse Functions |
| 8. Integration Tech. |
| 9. Integrate App. |
| 10. Parametric Eqs. |
| 11. Polar Coord. |
| 12. Series |
| Appendix |
| Basic Math |
| Units |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| |
||||||||||||
In this section the higher order derivatives of a function and the method to calculate them are discussed. |
||||||||||||
| Higher Order Derivatives |
||||||||||||
|
When more than one derivative is applied to a function, it is consider higher order derivatives. For example, the derivative of y = x7 is 7x6. 7x6 is differentiable and its derivative is 42x5. 42x5 is called the second order derivative of y with respect to x for function x7. Repeating this process, function y's third order derivative 210x4 is obtained. The higher order derivative notations is shown on the left. The chain rule also holds for higher order derivatives.
For instance, one can find the fourth derivative for Consider y is a function of θ where θ = ωt + 1. Therefore, the first order derivative is The second order derivative is The third order derivative is The forth order derivative is The implicit differentiation method also holds for higher
order derivatives. For example, one can find the second derivative for
ellipse
In ellipse, y is a function of x. In order to find the
derivative of y with respect to x, implicit differentiation method is
applied. The first derivative is Rearranging equation (1) gives Taking another derivative with respect to x gives Substitute equation (2) to (3), |
|||||||||||
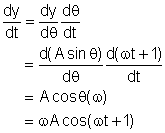
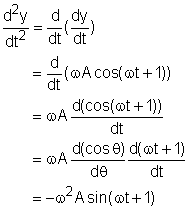
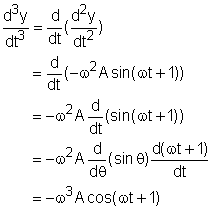
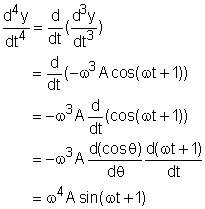
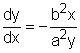 (2)
(2)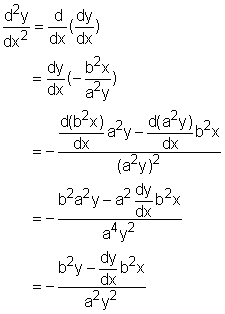 (3)
(3)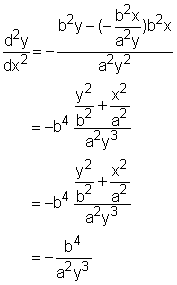 (4)
(4)