| Ch 2. Vectors | Multimedia Engineering Statics | ||||||
| Scalars & Vectors |
2-D Vectors |
3-D Vectors |
Dot Products |
||||
| Dot Products | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Vector Multiplication |
||
|
In the previous two sections, addition and subtraction of 2-D and 3-D vectors were illustrated. When "multiplying" two vectors, a special types of multiplication must be used, called the "Dot Product" and the "Cross Product". This section deals with only the dot product. The cross product is presented in a later section. |
||
| Dot Product |
||
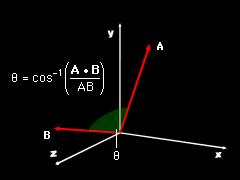 Dot Product Angle |
The dot product of two vectors A and B is defined as the product of the magnitudes A and B and the cosine of the angle θ between them: A • B = |A| |B| cosθ The dot product can also be calculated by A • B = Ax Bx + Ay By + Az Bz where vectors A and B are given as A = Ax i + Ay j + Az k |
|
| Applications of the Dot Product |
||
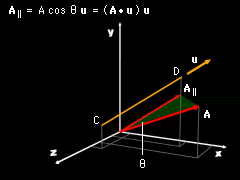 Component Parallel to a Line
|
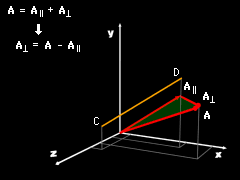
Using the dot product, the angle between two known vectors A and B, can be determined as If the direction of a line is defined by the unit vector u, then the scalar component of the vector A parallel to that line is given by A|| = A • u The vector component parallel to that line is given by A|| = (A • u) u Using the properties of vector addition, or the Pythagorean theorem, we can also determine the scalar and vector components of A perpendicular to the line: |
|
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.