| Ch 2. Vectors | Multimedia Engineering Statics | ||||||
| Scalars & Vectors |
2-D Vectors |
3-D Vectors |
Dot Products |
||||
| Dot Products | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Unit Vector Parallel to AB |
||
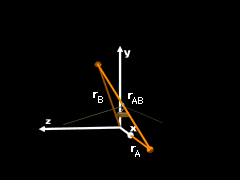 Adding rA and rB |
The direction of line AB is defined by the position vector rAB, which can be found from vector addition, rA + rAB
= rB = (39 - 175)i + (70 - 0)j + (29 - 0)k = -136i + 70j + 29k The unit vector in the direction of rAB can be determined by dividing rAB by its magnitude rAB, rAB = (-1,362 + 702 + 292)0.5 = 155.7 m uAB = rAB/rAB = -0.8736i + 0.4496j + 0.1863k |
|
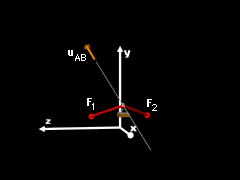 Total Force on Trolley |
||
| Total Force on Trolley |
||
|
Add the two forces applied to the trolley to find the total force, FT = F1 + F2 = -1,863i - 437.7j - 83.2k N |
||
| Component Parallel to uAB |
||
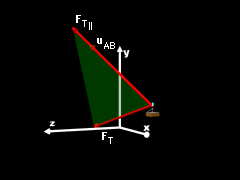 Force Component to Parallel to uAB |
The scalar component of the total force parallel to the direction uAB can be found by using the dot product, FT|| = FT • uAB = -1,863 (-0.8736) - 437.7 (0.4496) - 83.2 (0.1863) = 1,415 N This is just the magnitude of the total force acting in the direction of the cable AB. This can also be represented as a vector by multiplying this scalar component by the unit vector uAB, giving, FT|| = FT|| uAB = -1,236i + 636.3j + 263.7k N The magnitude or FT|| has not changed but is now represented as a vector. |
|
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.