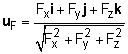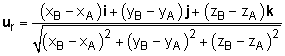| Ch 2. Vectors | Multimedia Engineering Statics | ||||||
| Scalars & Vectors |
2-D Vectors |
3-D Vectors |
Dot Products |
||||
| 3-D Vectors | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
|
|
Cartesian Components |
|
|
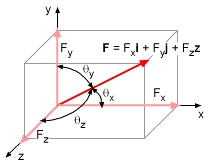
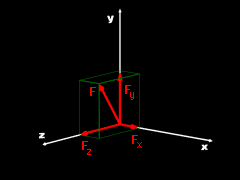
Recall, from the previous section, a vector in the x-y plane in can be written in Cartesian notation as, F = Fxi + Fyj Here i and j are unit vectors in the x and y directions. Likewise, a vector can be written in the three dimensional x-y-z space in Cartesian form, F = Fxi + Fyj + Fzk The new component, k is the unit vector in the z direction. The components Fx, Fy, and Fz can be determined from the magnitude and direction of F, Fx = F cosθx
The angle θi is the angle that F makes with the i axis as shown in the diagram at the top left.
|
||
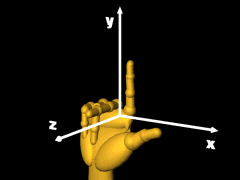 Right Hand Coordinate System |
Right-handed Coordinate System |
|
The order of the x-y-z coordinates does matter. If the three coordinates are aligned so that the thumb is in the x-direction when the first finger is in the y-direction and the second finger is in the z-direction, then it is called a right-handed coordinate system. Otherwise, it would be a left-handed coordinate system. Engineering always uses right-handed coordinate system. There is nothing wrong with a left-handed system, but all books, equations and technical papers use a right-handed system for convention. |
||
| Vector Addition |
||
|
|
Addition of 3-D vectors is the same as for 2-D except that three components must be added instead of two. If two vectors F1 and F2 exist, then the addition of F1 and F2 is, FR = F1+ F2 FR = (F1x + F2x)i + (F1y + F2y)j + (F1z + F2z)k The magnitude of the resultant vector is determined by applying the Pythagorean theorem, FR = ( FRx2 + FRy2 + FRz2 )0.5 The direction cosines are useful in some problems to identify each direction component. They are given as, cosθx = FRx /FR |
|
| 3D Unit Vector |
||
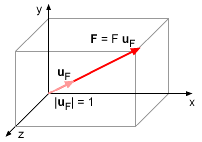 3-D Unit Vector |
General unit vectors can be determined in 3D using the definition of unit vectors, as uF = F/F Substituting vector notation and magnitude for F, gives This relationship is useful when the direction of the vector is needed. |
|
| Position Vectors |
||
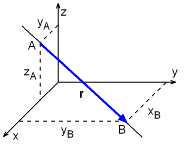 Position Vector |
In statics, force and moment vectors are the most commonly but many times a position vector is needed to help determine the direction of the force or moment vector. Position vectors are the same as all vectors, but they describe direction and distance, r = xi + yj + zk where x, y, and z are distances (scalars). Generally, position vectors are determined by its two end points, giving r = (xB - xA)i + (yB - yA)j + (zB - zA)k The directional unit vector for a position vector is ur = r/|r |
|
|
|
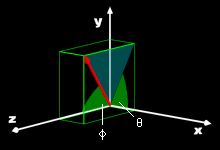
Spherical Coordinate Angles |
|
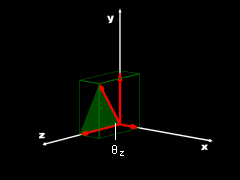
If the direction of the vector is defined by the two angles θ and φ, (shown at the left) as is commonly done in spherical coordinate systems, then the components are given by Fx = F sinφ cosθ The spherical angles θ and φ are given by, φ = cos-1 FRz /FR θ = sin-1 FRy /(FR sinφ) |
||
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.