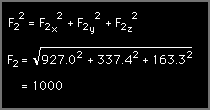| Ch 2. Vectors | Multimedia Engineering Statics | ||||||
| Scalars & Vectors |
2-D Vectors |
3-D Vectors |
Dot Products |
||||
| 3-D Vectors | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Normal Propeller (F1) |
||
 Normal Propeller Force Components |
With the x-y-z axes oriented as shown, the direction of the normal propeller is defined by the spherical angles θ and φ: θ1 = 175o The magnitude is F1 = 1000 lbs. The cartesian components can be calculated by, F1x = F sinφ1 cosθ1 Vector F1 can be written in Cartesian notation as F1 =-996.2i + 87.2j + 0k lb |
|
| Bent Propeller (F2) |
||
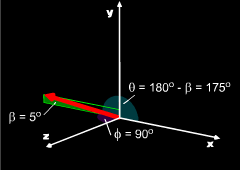
 Bent Propeller Force Components
|
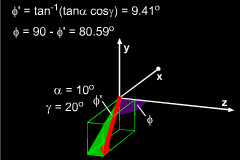
For the bent propeller, the spherical angles are more difficult to find but can be found from geometry. The animation at the left shows how to find these angles. They are, θ2 = 200o The magnitude F2 is given as 1000 lb. The component magnitudes are F2x = F sinφ2 cosθ2 = -927.0 lb Vector F2 can be written in Cartesian notation as F2 =-927.0i - 337.4j + 163.5k lb Next, add F1 and F2 to get the total force on the boat. FT = F1 + F2 = -1923.2i - 250.2j + 163.5k
|
|
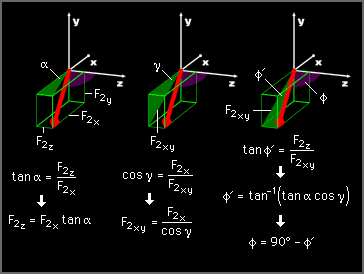 Angles for F2 |
||
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.