| Ch 2. Vectors | Multimedia Engineering Statics | ||||||
| Scalars & Vectors |
2-D Vectors |
3-D Vectors |
Dot Products |
||||
| Scalars, Vectors and Operations | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Algebraic Solution |
||
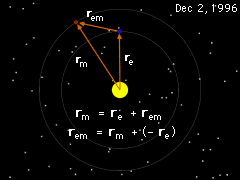
 Problem Diagram
|
The position vector from the Sun to Earth is re = re Ð 0o (counter-clockwise from vertical) = 150 × 106 km Ð 0o The position vector from the Sun to Mars: rm = rm Ð α = 207 × 106 km Ð 30o The difference between re and rm is the vector from Earth to Mars. Using the head-to-tail method gives, rm = re + rem This equation can be rearranged to give the unknown position vector rem, rem = rm
- re Subtracting the vectors, gives rem = 107.56 × 106 km
Ð 74.21o |
|
| Geometric Solution |
||
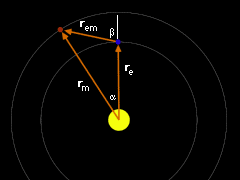
 Problem Distance and Angle |
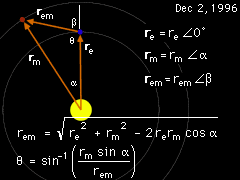
The problem can also be solved using pure geometry. First, define the position vector from Earth to Mars using the equation rm = rem Ð β Next, form a triangle with the three vectors and use the Law of the Cosine, rem = (re2 + rm2 - 2re rm cosα
)0.5 Then the internal angle θ, can be determined using the Law of the Sine, rem / sinα = rem / sinθ θ = sin-1 (rem sinα /rem ) = 105.79o Finally, the desired angle β is β = 180o - θ = 74.21o The position vector from Earth to Mars is rem = 107.56 × 106 km Ð 74.21o |
|
| Comments |
||
| This problem could also be solved using standard vector notation with unit vectors, i and j. Vector notation is introduced in the next several sections and is used extensively throughout Statics. | ||
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.