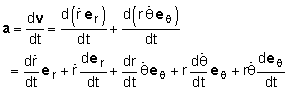| Ch 1. Particle General Motion | Multimedia Engineering Dynamics | ||||||
|
Position, Vel & Accel. |
Accel. vary w/ Time |
Accel. Constant | Rect. Coordinates | Norm/Tang. Coordinates | Polar Coordinates |
Relative Motion |
|
| Curv: Polar Coordinates | Case Intro | Theory | Case Solution | Example |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
|||||
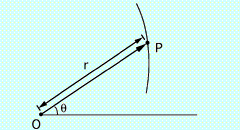 Position in Polar Coordinates |
For plane motion, many problems are better solved using polar coordinates, r and θ. This requires the development of position, velocity and acceleration equations based on, r and θ. |
||||
| Position |
|||||
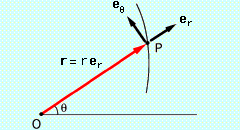 Units Vectors for Polar Coordinates |
Before velocity and acceleration can be determined in polar coordinates, position needs to be defined. Unlike rectilinear coordinates (x,y,z), polar coordinates move with the point and can change over time. Even though the r coordinate is moving, the position vector r, is measured in the r direction, giving
|
||||
| Velocity |
|||||
|
Similar to other coordinate systems, velocity can be determined by taking a time derivative of position, Since the coordinate system is moving, the time derivative of the unit vector, er, is not zero. Using a derivation similar to that found in the theory of n-t coordinate systems, expressions for the derivatives of the unit radial and unit transverse vectors can be determined as, The derivative of the eθ unit vector includes a negitve sign due to it changing inward as it moves (inward is a negative r-direction). The chain rule can then be used to express the time derivative of the unit radial vector as Substituting the above relationships into the velocity equation gives |
|||||
| Acceleration |
|||||
| A time derivative of the velocity will give an expression for the acceleration,
The chain rule can be used to express the time derivative of the unit transverse vector as By substituting this into the previous equation, and rearranging, gives the acceleration in terms of radial and transverse components, It is important to note that both r and θ directions have multiple terms. Acceleration in the r and θ direction is not just d2r/dt2 and d2θ/dt2, respectively, since the coordinates are moving. |
|||||
Practice Homework and Test problems now available in the 'Eng Dynamics' mobile app
Includes over 400 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.