| Ch 1. Particle General Motion | Multimedia Engineering Dynamics | ||||||
|
Position, Vel & Accel. |
Accel. vary w/ Time |
Accel. Constant | Rect. Coordinates | Norm/Tang. Coordinates | Polar Coordinates |
Relative Motion |
|
| Curv. Motion: Normal/Tangential | Case Intro | Theory | Case Solution | Example |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
|||||
|
In the Curvilinear Motion: Rectilinear Coordinates section, it was shown that velocity is always tangent to the path of motion, and acceleration is generally not. If the component of acceleration along the path of motion is known, motion in terms of normal and tangential components can be analyzed. |
|||||
 Motion of a Particle in a Plane |
Plane Motion of a Particle |
||||
|
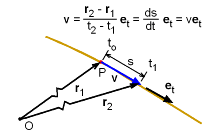
Consider a point P moving along a curved path. The position vector r specifies the position of P with respect to the reference point O, and s measures the position of P along the path relative to the reference point O'. The unit tangent vector, et, is tangent to the path at point P. |
|||||
| Velocity |
|||||
 Velocity of a Particle in a Plane |
The speed of the point along the path is found by taking the derivative of the position, v = ds/dt Since the velocity is tangent to the path, it can be expressed in terms of et, |
||||
| Acceleration |
|||||
|
|
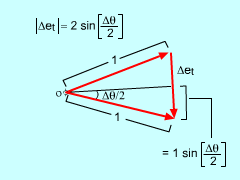
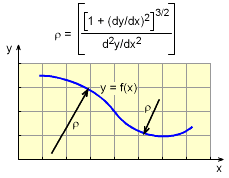
Similar to rectilinear coordinates, acceleration is obtained by differentiating the velocity (two parts) as a = dv/dt = dv/dt et + v det/dt Since the acceleration is not, in general, tangent to the path, it is useful to express it in terms of components that are normal and tangent to the path. To do so, the time derivative of the unit tangent vector, et, will be found. Let et(t) be the unit tangent vector at time t, and et(t + Δt) be the unit tangent vector at time t + Δt. If et(t) and et(t + Δt) are drawn from the same origin, they form two radii of length 1 on the unit circle. The magnitude of Δet is given by the equation Now, consider the vector Δ>et/Δθ. As Δθ approaches 0, Δet/Δθ becomes tangent to the unit circle (perpendicular to the path of P). The magnitude of Δet/Δθ< approaches 1. Next, define the unit normal vector as The chain rule can be used with the time derivative of the unit tangent vector to give But ds/dt = v, det/dθ = en, and dθ/ds = 1/ρ (where ρ is the radius of curvature). After substituting, the time derivative of the unit tangent vector becomes |
||||
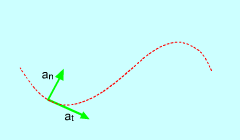 Normal and Tangential Acceleration |
The final equation for the acceleration in terms of both normal and tangential components is |
||||
Practice Homework and Test problems now available in the 'Eng Dynamics' mobile app
Includes over 400 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.